World Cup 2022 - Applying Statistics To Betting Odds #3

Hello there!

Being someone who has a keen interest in Statistics, it's always fun and interesting to put some of the knowledge into practice and real life applications. In this case, I am referring to betting odds.

But before I begin, I thought I should put out a big and bold disclaimer that this is solely a textbook practice and it's not meant to encourage anyone to bet because statistics is always about probabilities and my analysis is just meant to be a fun exercise (and this means I could be way wrong!).

This post is a continuation of the earlier post so you might want to refer to it for background.
Post #1
Post #2

---

Predicting Total Goals In World Cup 2022

How the tables have turned!

The last 8 matches saw only 16 goals, and the odds have greatly swung to a low scoring tournament.

But is it true? Let's take a look at the model.

---

Where We Are

After 40 matches:

• Total Goals: 97
• Mean (average goals per match): 2.43
• Variance: 3.69

As there are a total of 64 matches, we are more than halfway through and there are 24 matches to go. Using the data of 40 matches played as a sample, we can apply lambda (λ), or mean, of 2.43. So the mean goals for the remaining matches would be 24 * 2.43 = 58.20.

So we are assuming the remaining goals for 24 matches (X) would follow a Poisson distribution with mean 58.20, i.e. X ~ Po(58.20).

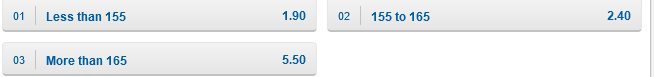
Looking back on the betting odds:

• If we needed the total goals to be less than 155, that means we can only afford to have 57 more goals given the current tally is 97. Punching the numbers into Excel/Google Sheet, P(X <= 57) = 47.21%.
• If we needed the total goals to be between 156 to 165, that means we want to have 58 to 68 more goals given the current tally is 97. Punching the numbers into Excel/Google Sheet, P(58 <= X <= 68) = 43.68%.
• If we needed the total goals to be more than 165, that means we want to have 69 or more goals given the current tally is 97. P(X >= 69) = 9.11%.

Presenting these numbers in a table and including the betting odds in the earlier screenshot:

Total Goals	Probability	Converting to Odds	Actual Odds
Less than 155 Goals	20.39% -> 47.21%	2.12	3.15 -> 1.90
155 to 165 Goals	45.32% -> 43.68%	2.29	1.95 -> 2.40
More than 165 Goals	34.29% -> 9.11%	10.98	3.35 -> 5.50

Comparing the odds by models vs the betting odds, there's not much value is any of the bets. I have earlier stuck my neck out and call for "More than 165 Goals". Like I mentioned in the earlier posts, the final 16 matches have a chance of additional 30 minutes of extra time if they end as a draw at full time. More minutes of playing means more chance for goals.

Of course, the same caution applies.

• The model is based on the assumption that the goals follow a Poisson Distribution. (Which might or might not be true)
• I have used the sample mean based on the current 40 matches, which might or might not be reflective of the full 64 matches. If you use a different mean, the results would be different.
• The mean and variance for the 40 matches have come down drastically but are slightly different for now, so a Poisson distribution might not be a strong fit. But I will observe as the matches go on.

In conclusion, I will stick to my earlier call and remain status quo for this update! Wish me luck!

With that, thanks for reading! Really appreciate it if you have gone this far. As usual, let me know any thoughts that you have!